Maurits
Cornelis Escher
Maurits Cornelis Escher, known as M. C. Escher, was a Dutch artist renowned for his mathematically-inspired prints. His work often featured impossible constructions, explorations of infinity, symmetry and tessellations, merging art with elements of mathematical theory. If you’re looking for Escher original prints and editions for sale or would like to sell, request a complimentary valuation and browse our network’s most in-demand works.
Maurits Cornelis Escher art for sale
Discover Maurits Cornelis Escher prints for sale, exclusively available through our private network of collectors. Explore signed and unsigned screenprints, lithographs, digital prints, and rare editioned proof prints by era-defining blue chip artists.
Sell Your Art
with Us
with Us
Join Our Network of Collectors. Buy, Sell and Track Demand
Biography
M. C. Escher was born on June 17, 1898, in Leeuwarden, Netherlands. He initially started architectural studies but quickly shifted to graphic arts at the School for Architecture and Decorative Arts in Haarlem, where he honed his skills in drawing and printmaking.
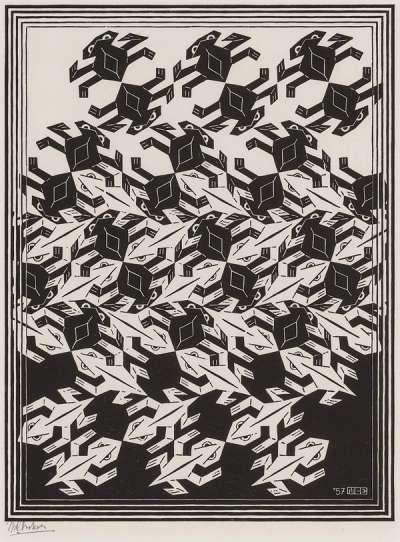
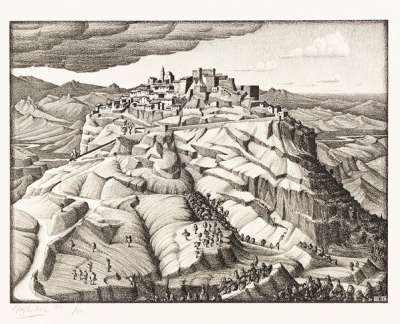
Escher's early works were predominantly landscapes, influenced by his extensive travels in the 1920s and 1930s, which profoundly shaped his artistic direction. The 1930s marked a significant shift in Escher's work from realism towards more abstract patterns and designs, and his creations began to feature impossible objects, explorations of infinity and complex geometric designs, blending art with mathematical concepts. His works often depicted impossible constructions, such as endless staircases and seamlessly interlocking spaces, challenging the viewers' perception of reality.
During and after World War II Escher gained popular fame, with his works appealing to art enthusiasts, scientists and mathematicians alike, and viewers attracted by the intricate themes in his work. Despite this, the formal art world often neglected his achievements, and it has only been in the 21st century that his works began to be widely exhibited. Escher's later years were spent in Baarn, Netherlands, where he continued to work despite illness. His exploration of complex patterns, optical illusions, and what he called 'mental imagery' continued to evolve until his death in 1972. Today, Escher's work remains hugely popular, showcased in exhibitions worldwide.
Escher’s early life was marked by a fascination with drawing and carpentry, where his artistic talents were evident. He was a sickly child, which affected his schooling, and he had to repeat the second grade. Despite this, he would go on to create some of the most complex compositions in 20th century art in his work: Escher had no formal training in mathematics, yet he possessed an intuitive understanding of the subject, which he keenly expressed through his pieces. He saw himself as more of a craftsman than a mathematician or scientist.
1922 was a pivotal year for Escher as he embarked on a journey through Italy, visiting various cities including Florence and Siena, before travelling to Spain, where he explored Madrid and Granada. The Moorish architecture of the Alhambra in Granada, particularly its geometrically symmetrical decorations, deeply impressed Escher. This exposure sparked his lifelong interest in tessellation, a mathematical concept involving interlocking patterns, which significantly influenced his artistic work. Escher moved to Rome in 1923, where he lived until 1935 and left due to the rise of Fascism. During this time, he married Jetta Umiker, a Swiss woman he met in Italy, and the couple had three sons. Escher's Italian period was marked by frequent travels to various locales such as Abruzzi, Corsica and Sicily. The landscapes, townscapes and colours of these regions prominently featured in his art. In 1936, Escher revisited Spain, spending extensive time at the Alhambra. He made detailed drawings of its mosaic patterns, demonstrating his growing fascination with tessellation, which became almost an obsession and played a crucial role in his artistic development.
Decades later, Escher’s work continues to influence artists, graphic designers and mathematicians. His legacy is that of an artist who transcended the conventional boundaries of art, making a unique and lasting imprint on both the art world and the realm of mathematical thought.